4.MD.C.7

In meeting CCSS in math, Code.org has a wonderful resource for 4th-grade math standard 4.MD.C.7 - Code with Anna & Elsa. For a hands-on...real-world application of this standard, please consider using this learning experience for your students!

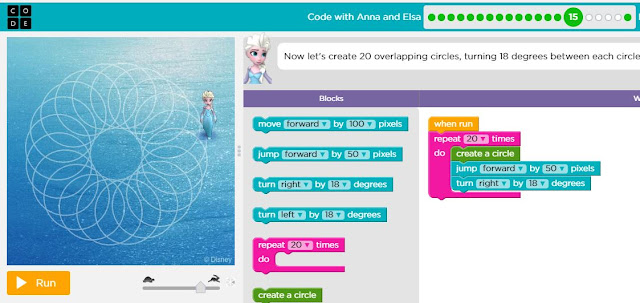
Code.org does a wonderful job in building the progression of student understanding. For example, in the standard and screen-shot below, students must understand that squares are made up of 4 equal sides and 90-degree angles in order to correctly complete puzzles 1-3.

This lesson also touches on 4.MD.6 Measure angles in whole-number degrees using a protractor. Sketch angles of specified measure.

Teachers should also point out that in puzzle 4, the repeat block is just like repeated addition, which is the same as multiplication. In this case...

Repeated Addition                                   Multiplication

move forward by 100 pixels                     move forward by 100 pixels
turn right by 90 degrees                          turn right by 90 degrees

                 +                                                        x 4

move forward by 100 pixels
turn right by 90 degrees

                +

move forward by 100 pixels
turn right by 90 degrees

                +

move forward by 100 pixels
turn right by 90 degrees

             

Puzzles 5 - 20 progress in complexity and ask the learner to apply knowledge of the standards below. 

4.MD.5.a  An angle is measured with reference to a circle with its center at the common endpoint of the rays, by considering the fraction of the circular arc between the points where the two rays intersect the circle. An angle that turns through 1/360 of a circle is called a "one-degree angle," and can be used to measure angles.

4.MD.5.b Define an n-degree angle as n 1-degree angles

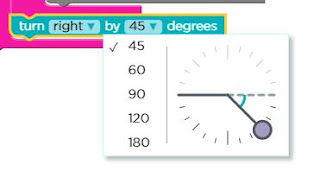
In each puzzle, students are provided with a protractor-type display that helps students determine which angle they may need to solve the puzzle.

THEN...they can explore...

4.MD.7 Recognize angle measure as additive. When an angle is decomposed into non-overlapping parts, the angle measure of the whole is the sum of the angle measures of the parts. Solve addition and subtraction problems to find unknown angles on a diagram in real world and mathematical problems, e.g., by using an equation with a symbol for the unknown angle measure.

In the measurement of angles, a composition is the joining of two angles as sums and is coded as addition. Likewise, decomposition describes the comparison of the measures (m) of two angles and is coded as subtraction. That is, for any angle <ABD located within

<ABC, m<ABD + m<DBC = m<ABC and m<ABC - m<ABD = m<DBC.

Rather than just completing a worksheet on 'missing angles', I would suggest 4th-grade teachers also use Code.org's "Code with Anna and Elsa." Students must find the measure of the angles that will add up to a complete circle = 360-degrees. It is important that an inquiry model of instruction is used, and Code.org suggests 'paired-programming' in which two students work together to complete the puzzles. In puzzle 8 below, students will more than likely use a trial-and-error method when determining that ten lines need to be etched in the ice. Therefore, each angle must be 36-degrees. 

For those who have purchased No Fear Coding: Computational Thinking Across the K-5 Curriculum, see page 86 for teacher - mathematical questions to ask. Otherwise, feel free to visit my TPT store and download a free copy of the "Code with Anna & Elsa - 4.MD.C.7 student worksheet.

Investing in Teacher Professional Development : Two Thumbs up for Governor Hutchinson!

Two thumbs up for Arkansas governor Hutchinson who has recognized this by allocating $800,000 in the state budget, targeted specifically for K-8 teacher training in the area of computer science.

I think we under-estimate how passionate our K-8 teachers are in joining this movement. They initially allowed for 250 teacher training slots, and these were filled up within the first week of the announcement. Hopefully, more states will follow suit...

About this article

Governor adds $300,000 to K-8 teacher computer science training stipends

The money will fund stipends for 150 teachers statewide who pursue the training, which begins this summer. The $300,000 announced Wednesday is…

ARKTIMES.COM

Careers with Code

This website provides educators with tools that can help students explore what they might be able to do with code. Careers with Code states, "We show how computer science can help young people create a dream career in any field, including health, sports, business, fashion, virtual reality and other exciting industries." The website hosts three main categories:

• What can I do with Code?
• How can I help others?
• Order the mag (free online viewing)

The 'Watch & Listen' tab contains career videos that are under two minutes and are appropriate for ANY age student. They are real people, doing real work, sharing their personal and professional lives. Videos from employees of Google, Code for America, Pixar, and NASA can be seen. They also have two 1/2 hour podcasts that are geared for 6-12th grade students.

The 'Educators' tab will take you to two Free downloadable Educator Resource files that contain activities on computational thinking, as well as computer science and social change. These resource books are meant to be used with the "Careers with Code" magazine. I conducted a readability test on Issue 2 2017/2018 edition and the Flesh-Kincaide Grade Level was 9.4. For those using F&P, that would equate to a level Z. The magazine is free to read online and is available to order in print.

GOLDEN NUGGET  = 'Get started tab'

This tab has a variety of short articles about CS+X. When you see CS+X this means Computer Science + any other subject area. They have articles about CS+Art, Social Goods, Sports, Sustainability, and Business. They even have a survey that asks: Which movie robot are you? 

Not sure what you will get...but it was determined that I was Wall-E.

Code Monkey = CS + Math

The CodeMonkey app website "is designed as a classroom resource with fully-guided lesson plans. This means that every teacher can teach the Codemonkey courses, even though they have no prior experience in Computer Science." 

The puzzles and games that were accessible in the free 7-day trial would easily allow any teacher to follow the sequences of tasks to gain a basic understanding of how to write code using a language called CoffeeScript. 

It should be noted that students using this app should have solid reading skills, as there are many directions that students must read with each task. 

While many of the courses are appropriate for upper elementary students, the 'Chat Course: Python Course" would be a challenge for those students who excel through the earlier courses.


GOLDEN NUGGET! 

In the free version, I was able to work through Part 1: Fundamentals and found these puzzles to be a great resource for 4th grade CCSS in math; specifically:

• 4.MD.A.1 - students need to use a ruler to measure the number of steps the monkey and turtle need to travel to collect the bananas
• 4.MD.C.6 -when students to measure angles in order to help the money arrive at its final destination (although not intuitive) the ruler can also be used as a protract to measure the angle the monkey needs to turn to cross the bridge in challenge #10